In this series of posts, I intend to consider financial derivatives as a whole and develop a conceptual framework in which any such derivative can be described. In doing so, I will focus on the information content of the relevant legal documents that is pertinent to the problem of calculating the derivative’s value. An important subsequent step is the development of the conceptual structure of the information that, while necessary for valuation, is not present in the contract. This leads us to our first and most important conceptual distinction that must underpin the design of a modern derivatives valuation platform:
A financial derivative is a legal agreement between a collection of (usually two) parties. The terms of the agreement are expressed in a document, the term sheet. As with any legal document, a term sheet expresses obligations and rights. The obligations relevant to the question of how much one party might be willing to pay for the privilege of entering such a contract, are those to make payments. Such payments might be of cash or of some other asset. The promise to pay, say, one dollar in a year’s time is perhaps the very simplest example of such a contract.
The rights relevant to a contract’s value are those to choose one course of action from a collection of many. The only aspects of the available courses of action relevant to the question of how much such a right is worth, are the obligations to make payments (and rights to make subsequent choices) contained within them. However, we have already associated the notion of “financial derivative contract” with such a collection of payment obligations and rights. It is clear that a recursive structure is emerging; we can model the valuation-specific aspects of a derivative contract by defining the concept of a Product as a collection of:
- obligations to make payments of cash or other assets and
- rights to other Products
(the name Product reflects the sell-side origin of the ideas developed in F3). For example, a Bermudan swaption represents a series of choices, each on a prescribed date. When the first choice arrives, the holder of such a contract has the right to enter an interest rate swap with a counterparty. The holder may choose not to enter such a contract at that time, in which case another opportunity to choose will arrive. Typically, such subsequent choices offer entry into the remainder of the same underlying swap, but this is not a requirement. We can see several nested Products here, illustrated below:
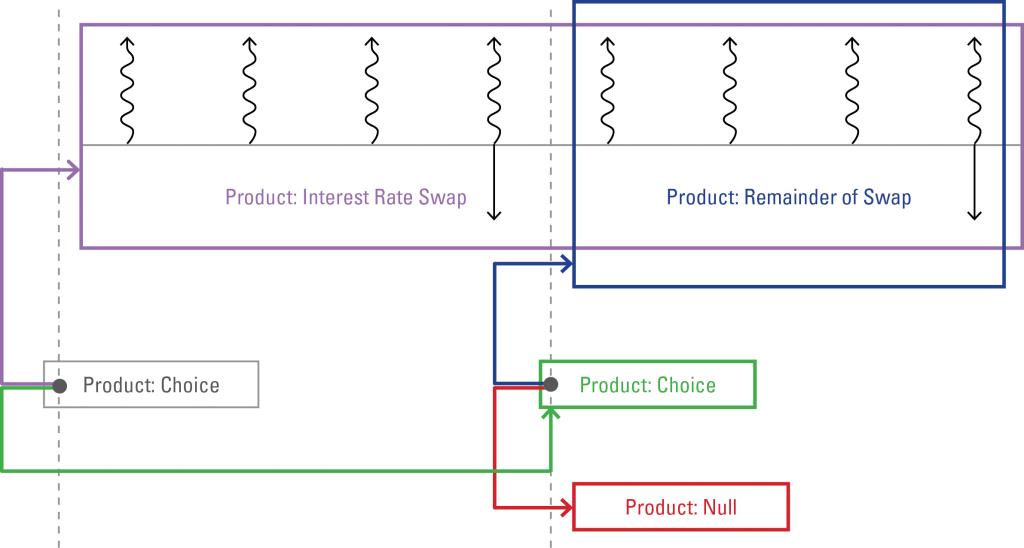
Schematic illustration of the Product structure of a Bermudan swaption.The Bermudan swaption itself is a Product, equivalent to the first choice, because all other relevant Products are contained within the two underlyings of that choice. One branch of the choice leads to an interest rate swap, another Product, but one consisting of only payment obligations, no rights to make choices. The other branch of the choice leads to a subsequent choice between the remainder of the same swap (another Product) and, because in this example the second choice is the last, nothing. Strictly, a contract devoid of any obligations or rights altogether is yet another Product, in the same way that zero is a number.
Next post: the (small number of) types of Product that form the building blocks of essentially all derivatives.

